The frick do you mean, "basically"? Is it or is it not?Does it suffice if my resulting calculations turn out to be "somewhat" correct too?

Natasha Nox 🇺🇦🇵🇸

The frick do you mean, "basically"? Is it or is it not?
Does it suffice if my resulting calculations turn out to be "somewhat" correct too?

Stuff like this confuses and stresses me out like hell. #math is supposed to be deterministic, at least at this point. Don't throw these wobbly words at me.

dianea 🏳️‍⚧️🦋🌱

@Natanox they used this excess wordy language in computer science textbooks and made reading even that very unenjoyable, compared to the technical books in the library, which were enjoyable to read, cover to cover, every day. Textbook language appears to be distinctive and designed to crush our spirits, especially when we come into the class and already have mastered the subject as self taught.

Natasha Nox 🇺🇦🇵🇸

@dianea I can tell you it's even worse if you're not a native english tongue. Any unspecific explanation has to go through yet another layer of abstraction.

@alan just told be how it's actually meant (more like in "fundamental, relating to", less like "somewhat, round about"). Still… I heavily dislike the way they put it.

dianea 🏳️‍⚧️🦋🌱

@Natanox @alan it's worse for me as that's the language style my narcissistic parent used before I was about to be subjected to trauma. That language over many years taught me to plan my exit from the situation to avoid severe abuse and the same patterns in a textbook was very stressful. Getting an engineering degree was very difficult when having to read this common unnecessary textbook language style.

Natasha Nox 🇺🇦🇵🇸

@dianea That sucks. For my part I'm just happy for all these resources to be available on the internet (even if it's not my native language). Given my school time was a nightmare I couldn't go to one again even if I had the means to. Learning in perceived safety at home gives me the possibility to work towards *something*.

remcycles

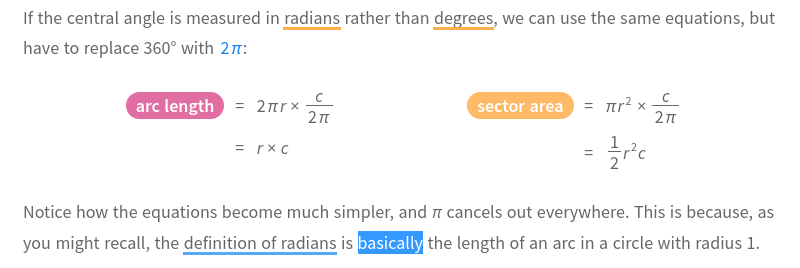
@Natanox "basically" isn't the problem. The "definition" isn't really a definition. As written, the arc could be any length. There's no language to link the arc length to the radius, which are equal for radians.

"The measure of a central angle subtending an arc equal in length to the radius." https://www.collinsdictionary.com/dictionary/english/radian

I highly recommend "The Tau Manifesto" for understanding radians and the unit circle better:
https://tauday.com/tau-manifesto#sec-circles_and_angles

Natasha Nox 🇺🇦🇵🇸

@remcycles Thank you very much for that tauday.com link. That's finally some explanation that furthers understanding.

I honestly don't understand why explanations are always "cut off" and hidden behind vague language (i.e. "it's basically" or "it's about as"). Sure I invested some time into looking into this today (and all the awesome answers I got after my little rant), but now I got a more intrinsic understanding of the whole concept and can work with it more naturally instead of abstract.

Andreas, DJ3EI, he/him

An arc length is - well, a length. Incidently, so is a radius. A length would be measured im m or inches or miles or what have you.

An angle is not a length. It is measured in degrees or radians.

So to say "by definition, radians is an arc length" is just a bit imprecise. That "basically" has a reason.

It would be a different story if those textbook authors could somewhere find a circle with radius 1. Not 1 m or 1 inch or 1 mile, but just 1.

@Natanox
@dianea

Tobias Denkinger

@dj3ei
A mathematical length can be measured in any unit.

If, for example, you measure the length of a line between two points on a time axis, your length will be in a unit of time (not a unit of distance).

Similarly, if you measure the length of an arc on the unit circle (which has radius 1, not 1 meter or 1 inch), the unit of length will be 1 and not any unit of distance.

The properties of mathematical objects are independent of how you represent them on a piece of paper.
@Natanox @dianea

Natasha Nox 🇺🇦🇵🇸

@denki @dj3ei @dianea This was what made it understandable to me.
https://tauday.com/tau-manifesto#sec-circles_and_angles

The reason for why the writer wrote "basically" really is pointless for me to know unless it's about how the stuff they write about works.

> "The properties of mathematical objects are independent of how you represent them on a piece of paper."

That somewhat touches why the "basically" confused me so much. The "basically" obfuscates the actual properties I needed to learn, especially in their sentence.

Natasha Nox 🇺🇦🇵🇸

@denki @dj3ei @dianea
What I mean by that: By saying "it's basically like that in this specific context", it leaves open whether or not it might be different in other contexts. Perhaps radians have inherent scaling properties? Could be, the way it's put up there. Perhaps it's just an approximation and there's no logical connection between Arc length and radians at all and it just happens to be comparable in a unit circle.

Learning mathematics shouldn't be about interpreting the teacher.